Lecture 8: Manifolds

8.1 Definition of Manifolds, fields and forms

Throughout this lecture, the word differentiable will be used to mean manifolds1.png .

Definition 1: A diffeomorphism is a function manifolds2.png between open sets of manifolds3.png which is differentiable and has a differentiable inverse.

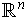Definition 2: The half-space manifolds4.png is the set of points in manifolds5.png whose last coordinate is non-negative. A k-dimensional manifold-with-boundary is a subset manifolds6.png of manifolds7.png such that every point manifolds8.png satisfies:

There is a diffeomorphism manifolds9.png from an open neighborhood of manifolds10.png such that

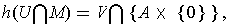
manifolds11.png

where the set 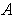manifolds12.png is either 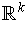manifolds13.png or manifolds14.png

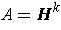The set of manifolds15.png which satisfy the condition with manifolds16.png is called the boundary of manifolds17.png and is denoted manifolds18.png If the boundary of manifolds19.png is empty, then manifolds20.png can be referred to as a manifold.

If manifolds21.png , then a coordinate system around manifolds22.png is a 1-1 differentiable function manifolds23.png for which there is an open set manifolds24.png satisfying:

  1. manifolds25.png
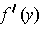  2. manifolds26.png has rank 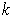manifolds27.png for each manifolds28.png
  3. manifolds29.png is continuous.

Proposition 1: A subset manifolds30.png of manifolds31.png is a k-dimensional manifold if and only if every manifolds32.png has a coordinate system about manifolds33.png

Proof: If manifolds34.png has a function manifolds35.png as in the definition of manifold, then let manifolds36.png be the projection on the first manifolds37.png coordinates of manifolds38.png and manifolds39.png be defined by manifolds40.png Then manifolds41.png is a coordinate system about manifolds42.png . (The condition involving the rank follows by applying the chain rule to manifolds43.png where manifolds44.png is with manifolds45.png being the projection on the first manifolds46.png coordinates.)

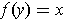Conversely, if manifolds47.png is a coordinate system about manifolds48.png , then define manifolds49.png by manifolds50.png . If manifolds51.png is such that manifolds52.png , then manifolds53.png is invertible in a neighborhood of manifolds54.png . The inverse in this neighborhood is the desired function manifolds55.png as in the definition of manifold.

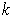Note: If manifolds56.png and manifolds57.png are a coordinate systems about manifolds58.png , then manifolds59.png is a diffeomorphism as the proof showed that manifolds60.png is just the first manifolds61.png coordinates of the diffeomorphism manifolds62.png

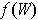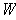In particular, manifolds63.png is independent of the choice of coordinate system manifolds64.png about manifolds65.png This set is called the tangent space of manifolds66.png at manifolds67.png If manifolds68.png is a element of the tangent space at manifolds69.png for every manifolds70.png in manifolds71.png , then there is a unique manifolds72.png in the tangent space of manifolds73.png at manifolds74.png such that manifolds75.png We say manifolds76.png is a differentiable vector field of manifolds77.png if each of the manifolds78.png are differentiable vector fields of each manifolds79.png . In particular, if manifolds80.png is the restriction of a differentiable vector field on some open subset manifolds81.png containing manifolds82.png , then manifolds83.png restricts to a differentiable vector field on manifolds84.png

Similarly, a differentiable k-form on manifolds85.png is a function manifolds86.png which assigns to each manifolds87.png an manifolds88.png such that manifolds89.png is a differentiable k-form on manifolds90.png for every coordinate system manifolds91.png about manifolds92.png . The derivative manifolds93.png of manifolds94.png is the differentiable k-form on manifolds95.png provided by the following result:

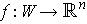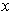Proposition 2: If manifolds96.png is a differentiable k-form on manifolds97.png , then there is a unique differentiable manifolds98.png -form on manifolds99.png such that for every coordinate system manifolds100.png about manifolds101.png , one has

manifolds102.png

Proof: For each manifolds103.png , let manifolds104.png be chosen so that manifolds105.png . Let

manifolds106.png

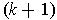This definition is independent of the choice of coordinate system manifolds107.png and is easily shown to be the desired differentiable manifolds108.png -form on manifolds109.png

8.2 Orientation and Integrals

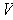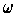Let manifolds110.png be a finite dimensional real vector space of dimension manifolds111.png and manifolds112.png be non-zero. Then manifolds113.png is non-zero for every basis manifolds114.png of manifolds115.png . Thus, the set of (ordered) bases of manifolds116.png is partitioned into two sets, such that two bases are in the same set if and only if manifolds117.png applied to the bases gives a real number of the same sign. 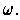The set to which an (ordered) basis belongs is called its orientation and is denoted manifolds118.png Note that two bases being of the same orientation is independent of the choice of manifolds119.png The usual orientation of manifolds120.png is defined to be manifolds121.png

Suppose that for every manifolds122.png (where manifolds123.png is a k-dimensional manifold), one has chosen manifolds124.png an orientation of the tangent space manifolds125.png . Then these choices are said to be consistent if and only if for every coordinate system manifolds126.png about manifolds127.png and every pair manifolds128.png , one has manifolds129.png if and only if manifolds130.png Such a consistent choice is called an orientation of manifolds131.png ; a manifold which admits an orientation is said to be orientable.

If the manifolds132.png are consistent, then one says that the coordinate system manifolds133.png is said to be orientation preserving if manifolds134.png for every manifolds135.png Clearly, if manifolds136.png is a linear transformation with manifolds137.png , then exactly one of manifolds138.png and manifolds139.png is orientation preserving.

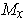Now, let manifolds140.png be a manifolds141.png -dimensional manifold-with-boundary and manifolds142.png Then manifolds143.png is a manifolds144.png -dimensional subspace of manifolds145.png . There are precisely two unit vectors perpendicular to this subspace. Choose a coordinate system manifolds146.png about manifolds147.png in which 0 maps to manifolds148.png and manifolds149.png Then the one of the unit vectors of the form manifolds150.png with manifolds151.png is called the outward unit normal manifolds152.png Suppose we have an orientation manifolds153.png for manifolds154.png . Then choose manifolds155.png a basis for manifolds156.png such that manifolds157.png . Then the manifolds158.png define a consistent orientation on manifolds159.png called the induced orientation. 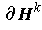Note that the orientation induced on manifolds160.png from the usual orientation on manifolds161.png is the usual orientation if and only if manifolds162.png is even.

If manifolds163.png is manifolds164.png -dimensional manifold contained in manifolds165.png which admits an orientation manifolds166.png , then one can also define an outward unit normal manifolds167.png as the one such that if manifolds168.png is a basis of manifolds169.png with manifolds170.png , then manifolds171.png is the usual orientation of manifolds172.png

If manifolds173.png is a manifolds174.png -form on a manifolds175.png -dimensional manifold-with-boundary manifolds176.png and manifolds177.png is a singular manifolds178.png in manifolds179.png , we can define:

manifolds180.png

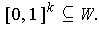Integrals over manifolds181.png -chains are defined in the obvious way. In the special case where manifolds182.png , we will always assume that manifolds183.png is the restriction to manifolds184.png of a coordinate system manifolds185.png (where we assume manifolds186.png If one has an orientation for manifolds187.png , we say that the singular manifolds188.png -cube is orientation preserving provided that manifolds189.png is.

All the definitions have been set up to guarantee the following result:

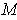Proposition 3: If manifolds190.png are two orientation preserving singular manifolds191.png -cubes in an oriented manifolds192.png -dimensional manifold manifolds193.png and manifolds194.png is a manifolds195.png -form on manifolds196.png such that manifolds197.png outside of manifolds198.png , then

manifolds199.png

Proof: We have

manifolds200.png

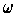where manifolds201.png and we have used the assumption that manifolds202.png is zero outside of manifolds203.png It remains to show that

manifolds204.png

But, if manifolds205.png , then we have

manifolds206.png

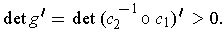since manifolds207.png The result now follows by the change of variables formula for integrals.

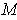We can now define integrals. Let manifolds208.png be a k-form on an oriented k-dimensional manifold manifolds209.png . Choose an open cover manifolds210.png of manifolds211.png such that for each manifolds212.png , there is an orientation preserving singular manifolds213.png -cube manifolds214.png with manifolds215.png Let manifolds216.png be a partition of unity for manifolds217.png subordinate to this cover. Define

manifolds218.png

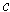where manifolds219.png was chosen so that manifolds220.png is zero outside of an compact subset of manifolds221.png and manifolds222.png is is an orientation preserving singular manifolds223.png -cube with manifolds224.png Then just as in Chapter 3, the value of this integral is independent of the choice of manifolds225.png , manifolds226.png , and manifolds227.png .

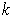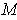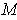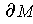Now suppose we have a manifolds228.png -dimensional manifold-with-boundary manifolds229.png with orientation manifolds230.png . Let manifolds231.png be the orientation induced by manifolds232.png on manifolds233.png . Let manifolds234.png be an orientation-preserving manifolds235.png -cube in manifolds236.png such that manifolds237.png lies in manifolds238.png and this is the only face which contains any interior points of manifolds239.png . 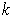Then manifolds240.png is orientation preserving if and only manifolds241.png is even. In particular, if manifolds242.png is a manifolds243.png -form on manifolds244.png which is zero outside of manifolds245.png , we have

manifolds246.png

Now manifolds247.png appears with coefficient manifolds248.png in the definition of manifolds249.png . So,

manifolds250.png

This explains the strange choice of signs in the definition of the induced orientation on manifolds251.png

8.3 Stokes' Theorem for Manifolds-with-Boundary

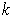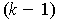Theorem 1: (Stokes' Theorem) Let manifolds252.png be a compact oriented manifolds253.png -dimensional manifold-with-boundary and manifolds254.png be a manifolds255.png -form on manifolds256.png . Then

manifolds257.png

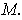
where manifolds258.png is oriented with the orientation induced from that of manifolds259.png

Proof: Begin with two special cases: First assume that there is an orientation preserving manifolds260.png -cube in manifolds261.png such that manifolds262.png outside of manifolds263.png Using our earlier Stokes' Theorem, we get

manifolds264.png

since manifolds265.png on manifolds266.png But manifolds267.png also since manifolds268.png on manifolds269.png

The second case is where there is an orientation-preserving singular manifolds270.png -cube in manifolds271.png such that manifolds272.png is the only face containing points of manifolds273.png and manifolds274.png outside of manifolds275.png One has:

manifolds276.png

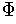For the general case, choose an open cover manifolds277.png of manifolds278.png and a partition of unity manifolds279.png subordinate to manifolds280.png such that for each manifolds281.png , the form manifolds282.png is as in one of the two cases already considered. Since manifolds283.png is compact, one has a finite sum:

manifolds284.png

But then,

manifolds285.png

8.4 Volume

In manifolds286.png , the volume can be calculated as the integral of the form manifolds287.png . We would like to find a generalization to manifolds of this differential form.

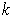If manifolds288.png is a manifolds289.png -dimensional manifold, then the usual inner product on manifolds290.png induces an inner product on each of the tangent spaces of manifolds291.png . (Recall that an inner product of V is a bilinear form manifolds292.png such that manifolds293.png for all manifolds294.png .) With an inner product, one can define an orthonormal basis to be one of the form manifolds295.png where manifolds296.png where manifolds297.png is the Kronecker manifolds298.png . Now, if manifolds299.png and manifolds300.png are both orthonormal bases, then we can write manifolds301.png . In particular, one calculates:

manifolds302.png

which can be expressed as a matrix equation manifolds303.png where manifolds304.png is the matrix with entries manifolds305.png Taking determinants of this means that manifolds306.png In particular, if manifolds307.png where manifolds308.png is a vector space of dimension manifolds309.png , then

Proposition 4: manifolds310.png is constant for all orthonormal bases of manifolds311.png of the same orientation.

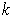Definition 3: Let manifolds312.png be an oriented manifolds313.png -dimensional manifold in manifolds314.png . Then a manifolds315.png -form manifolds316.png on manifolds317.png is called a volume element if manifolds318.png for all orientation preserving orthonormal bases manifolds319.png

Example: Consider the case of 2-dimensional oriented manifolds in 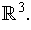manifolds320.png Let manifolds321.png be the outward normal at manifolds322.png . Then define manifolds323.png by

manifolds324.png

By the definition of the outward normal, manifolds325.png is a volume element. Further, if manifolds326.png is an orthonormal basis of the same orientation as manifolds327.png , one has:

manifolds328.png

Also, expanding as cofactors of the last row, one gets

manifolds329.png

On manifolds330.png , one can compute for manifolds331.png using manifolds332.png for some manifolds333.png , that

manifolds334.png

Letting manifolds335.png , and manifolds336.png , we get:

Proposition 4: Let manifolds337.png be an oriented 2-dimensional manifold in manifolds338.png and let manifolds339.png be the unit outward normal. Then the volume element manifolds340.png satisfies:

manifolds341.png

Further, on manifolds342.png , one has:

manifolds343.png

To calculate a surface area, we need to evaluate manifolds344.png for an orientation preserving singular 2-cube manifolds345.png . The integrand is

manifolds346.png

where

manifolds347.png

(See Problem 4.9, part e.)

8.4 Classical Stokes' Theorem

Three separate results will be shown to be special cases of our Stokes' Theorem.

Theorem 2: (Green's Theorem) Let manifolds348.png be a compact 2-dimensional manifold-with-boundary. Suppose that manifolds349.png are differentiable. Then

manifolds350.png

Proof: This is just Stoke's Theorem in the case of a 1-form.

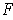Theorem 3: (Divergence Theorem) Let manifolds351.png be a compact 3-dimensional manifold-with-boundary and manifolds352.png be the unit outward normal on manifolds353.png . Let manifolds354.png be a differentiable vector field on manifolds355.png Then

manifolds356.png

In terms of manifolds357.png , this amounts to:

manifolds358.png

Proof: Define manifolds359.png Then manifolds360.png Further, Proposition 4 says that:

manifolds361.png

So, we see that this is also a special case of Stokes' Theorem

Theorem 4: (Stokes' Theorem) Let manifolds362.png be a compact oriented 2-dimensional manifold-with-boundary and manifolds363.png be the unit outward normal on manifolds364.png determined by the orientation on manifolds365.png . Let manifolds366.png have the induced orientation. Let manifolds367.png be the vector field on manifolds368.png with manifolds369.png and manifolds370.png be a differentiable vector field in an open set containing manifolds371.png . Then

manifolds372.png

In terms of manifolds373.png , this amounts to:

manifolds374.png

Proof: Let manifolds375.png on manifolds376.png be defined by manifolds377.png Again, using Proposition 4, we get:

manifolds378.png

Since manifolds379.png , one has

manifolds380.png

as one can see by evaluating each equation at manifolds381.png . It follows that

manifolds382.png

So, this is also a special case of our earlier Stokes' Theorem.