Exercises: Chapter 2, Section 5

  1. Let ch2e1.png be an open set and ch2e2.png a continusously differentiable 1-1 function such that ch2e3.png for all ch2e4.png . Show that ch2e5.png is an open set and ch2e6.png is differentiable. Show also that ch2e7.png is open for any open set ch2e8.png .

    For every ch2e9.png , there is an ch2e10.png with ch2e11.png . By Theorem 2-11, there is an open set ch2e12.png and an open subset ch2e13.png such that ch2e14.png and ch2e15.png . Since clearly ch2e16.png , this shows that ch2e17.png is open. Furthermore ch2e18.png is differentiable. It follows that ch2e19.png is differentiable at ch2e20.png . Since ch2e21.png was arbitrary, it follows that ch2e22.png is differentiable.

    By applying the previous results to the set ch2e23.png in place of ch2e24.png , we see that ch2e25.png is open.

    1. Let ch2e26.png be a continuously differentiable function. Show that ch2e27.png is not 1-1.

      We will show the result is true even if ch2e28.png is only defined in a non-empty open subset of ch2e29.png . Following the hint, we know that ch2e30.png is not constant in any open set. So, suppose we have ch2e31.png (the case where ch2e32.png is analogous). Then there is an open neighborhood ch2e33.png of ch2e34.png with ch2e35.png for all ch2e36.png . The function ch2e37.png defined by ch2e38.png satisfies ch2e39.png for all ch2e40.png . Assuming that ch2e41.png and hence ch2e42.png are 1-1, we can apply Problem 2-36. The inverse function is clearly of the form ch2e43.png and so ch2e44.png for all ch2e45.png . 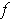Now ch2e46.png is open but each horizontal line intersects ch2e47.png at most once since ch2e48.png is 1-1. This is a contradiction since 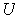ch2e49.png is non-empty and open.

    2. Generalize this result to tthe case of a continuously differentiable function ch2e50.png with ch2e51.png .

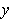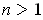      By replacing ch2e52.png with a vector of variables, the proof of part (a) generalizes to the case where ch2e53.png is a function defined on an open subset ch2e54.png of ch2e55.png where ch2e56.png .

      For the general case of a map ch2e57.png where ch2e58.png is an open subset of ch2e59.png with ch2e60.png , if ch2e61.png is constant in a non-empty open set ch2e62.png , then we replace ch2e63.png with ch2e64.png and drop out ch2e65.png reducing the value of ch2e66.png by one. On the other hand, if ch2e67.png for some ch2e68.png , then consider the function ch2e69.png defined by ch2e70.png . Just as in part (a), this will be invertible on an open subset of ch2e71.png and its inverse will look like ch2e72.png . Replace ch2e73.png with ch2e74.png . 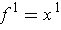Note that we have made ch2e75.png . Again, by restricting to an appropriate rectangle, we can simply fix the value of ch2e76.png and get a 1-1 function defined on on a rectangle in one less dimension and mapping into a space of dimension one less. By repeating this process, one eventually gets to the case where ch2e77.png is equal to 1, which we have already taken care of.

    1. If ch2e78.png satisfies ch2e79.png for all ch2e80.png , show that ch2e81.png is 1-1 on all of ch2e82.png .

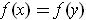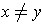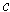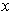      Suppose one has ch2e83.png for some ch2e84.png . By the mean value theorem, there is a ch2e85.png between ch2e86.png and ch2e87.png such that ch2e88.png . Since both factors on the right are non-zero, this is impossible.

    2. Define ch2e89.png by ch2e90.png . Show that ch2e91.png for all ch2e92.png but ch2e93.png is not 1-1.

      Clearly, ch2e94.png for all ch2e95.png . The function is not 1-1 since ch2e96.png for all ch2e97.png .

  4. Use the function ch2e98.png defined by

    ch2e99.png

    to show that continuity of the derivative cannot be eliminated from the hypothesis of Theorem 2-11.

    Clearly, ch2e100.png is differentiable for ch2e101.png . At ch2e102.png , one has

    ch2e103.png

    So ch2e104.png satisfies the conditions of Theorem 2-11 at ch2e105.png except that it is not continuously differentiable at 0 since ch2e106.png for ch2e107.png .

    Now ch2e108.png and it is straightforward to verify that ch2e109.png for all sufficiently large positive integers ch2e110.png . By the intermediate value theorem, there is a ch2e111.png between ch2e112.png and ch2e113.png where ch2e114.png . By taking n larger and larger, we see that ch2e115.png is not 1-1 on any neighborhood of 0.