Exercises: Chapter 2, Section 4

  1. Find expressions for the partial derivatives of the following functions:
    1. ch2d1.png

      ch2d2.png

    2. ch2d3.png

      ch2d4.png

    3. ch2d5.png

      ch2d6.png , ch2d7.png , ch2d8.png .

    4. ch2d9.png

      ch2d10.png

  2. Let ch2d11.png . For ch2d12.png , the limit

    ch2d13.png

    if it exists, is denoted ch2d14.png and called the directional derivative of ch2d15.png at ch2d16.png , in the direction ch2d17.png .

    1. Show that ch2d18.png .

      This is obvious from the definitions.

    2. Show that ch2d19.png .

      ch2d20.png

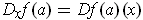    3. If ch2d21.png is differentiable at ch2d22.png , show that ch2d23.png and therefore ch2d24.png .

      One has

      ch2d25.png

      which shows the result whenever ch2d26.png . The case when ch2d27.png is trivially true.

      The last assertion follows from the additivity of the function ch2d28.png .

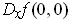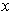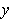  3. Let ch2d29.png be defined as in Problem 2-4. Show that ch2d30.png exists for all ch2d31.png , but if ch2d32.png , then ch2d33.png is not true for all ch2d34.png and all ch2d35.png .

    With the notation of Problem 2-4, part (a) of that problem says that ch2d36.png exists for all ch2d37.png . Now suppose ch2d38.png . Then ch2d39.png , But ch2d40.png .

  4. Let ch2d41.png be defined as in Problem 1-26. Show that ch2d42.png exists for all ch2d43.png , although ch2d44.png is not even continuous at (0,0).

    By Problem 1-26 (a), ch2d45.png for all ch2d46.png .

    1. Let ch2d47.png be defined by

      ch2d48.png

      Show that ch2d49.png is differentiable at 0 but ch2d50.png is not continuous at 0.

      Clearly, ch2d51.png is differentiable at ch2d52.png . At ch2d53.png , one has ch2d54.png since ch2d55.png .

      For ch2d56.png , one has ch2d57.png . The first term has limit 0 as ch2d58.png approaches 0. But the second term takes on all values between -1 and 1 in every open neighborhood of ch2d59.png . So, ch2d60.png does not even exist.

    2. Let ch2d61.png be defined by

      ch2d62.png

      Show that ch2d63.png is differentiable at (0,0) but that ch2d64.png is not continuous at ch2d65.png .

      The derivative at (0, 0) is the zero linear transformation because ch2d66.png , just as in part (a).

      However, ch2d67.png for ch2d68.png where ch2d69.png is as in part (a). It follows from the differentiability of ch2d70.png , that ch2d71.png and ch2d72.png are defined for ch2d73.png . (The argument given above also shows that they are defined and 0 at ch2d74.png .) Further the partials are equal to ch2d75.png up to a sign, and so they cannot be continuous at 0.

  6. Show that the continuity of ch2d76.png at ch2d77.png may be eliminated from the hypothesis of Theorem 2-8.

    Proceed as in the proof of Theorem 2-8 for all ch2d78.png . In the ch2d79.png case, it suffices to note that ch2d80.png follows from the definition of ch2d81.png . This is all that is needed in the rest of the proof.

  7. A function ch2d82.png is homogeneous of degree ch2d83.png if ch2d84.png for all ch2d85.png and ch2d86.png . If ch2d87.png is also differentiable, show that

    ch2d88.png

    Applying Theorem 2-9 to 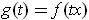ch2d89.png gives ch2d90.png . On the other hand, ch2d91.png and so ch2d92.png . Substituting ch2d93.png in these two formulas show the result.

  8. If ch2d94.png is differentiable and ch2d95.png , prove that there exist ch2d96.png such that

    ch2d97.png

    Following the hint, let ch2d98.png . Then ch2d99.png . On the other hand, Theorem 2-9 gives ch2d100.png . So, we have the result with ch2d101.png .