Exercises Chapter 2, Section 1

  1. Prove that if ch2a1.png is differentiable at ch2a2.png , then it is continuous at ch2a3.png .

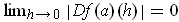    If ch2a4.png is differentiable at ch2a5.png , then ch2a6.png . So, we need only show that ch2a7.png , but this follows immediately from Problem 1-10.

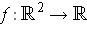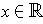  2. A function ch2a8.png is said to be independent of the second variable if for each ch2a9.png we have ch2a10.png for all ch2a11.png . Show that ch2a12.png is independent of the second variable if and only if there is a function ch2a13.png such that ch2a14.png . What is ch2a15.png in terms of ch2a16.png ?

    The first assertion is trivial: If ch2a17.png is independent of the second variable, you can let ch2a18.png be defined by ch2a19.png . Conversely, if ch2a20.png , then ch2a21.png .

    If ch2a22.png is independent of the second variable, then ch2a23.png because:

    ch2a24.png

    Note: Actually, ch2a25.png is the Jacobian, i.e. a 1 x 2 matrix. So, it would be more proper to say that ch2a26.png , but I will often confound ch2a27.png with ch2a28.png , even though one is a linear transformation and the other is a matrix.

  3. Define when a function ch2a29.png is independent of the first variable and find ch2a30.png for such ch2a31.png . Which functions are independent of the first variable and also of the second variable?

    The function ch2a32.png is independent of the first variable if and only if ch2a33.png for all ch2a34.png . Just as before, this is equivalent to their being a function ch2a35.png such that ch2a36.png for all ch2a37.png . An argument similar to that of the previous problem shows that ch2a38.png .

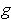  4. Let ch2a39.png be a continuous real-valued function on the unit circle 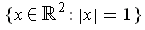ch2a40.png such that ch2a41.png and ch2a42.png . define ch2a43.png by

    ch2a44.png

    1. If ch2a45.png and ch2a46.png is defined by ch2a47.png , show that ch2a48.png is differentiable.

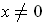      One has ch2a49.png when ch2a50.png and ch2a51.png otherwise. In both cases, ch2a52.png is linear and hence differentiable.

    2. Show that ch2a53.png is not differentiable at (0, 0) unless ch2a54.png .

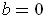      Suppose ch2a55.png is differentiable at ch2a56.png with, say, ch2a57.png . Then one must have: ch2a58.png . But ch2a59.png and so ch2a60.png . Similarly, one gets ch2a61.png . More generally, using the definition of derivative, we get for fixed ch2a62.png : ch2a63.png . But ch2a64.png , and so we see that this just says that ch2a65.png for all ch2a66.png . Thus ch2a67.png is identically zero.

  5. Let ch2a68.png be defined by

    ch2a69.png

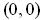    Show that ch2a70.png is a function of the kind considered in Problem 2-4, so that ch2a71.png is not differentiable at ch2a72.png .

    Define ch2a73.png by ch2a74.png for all ch2a75.png . Then it is trivial to show that ch2a76.png satisfies all the properties of Problem 2-4 and that the function ch2a77.png obtained from this ch2a78.png is as in the statement of this problem.

  6. Let ch2a79.png be defined by ch2a80.png . Show that ch2a81.png is not differentiable at 0.

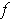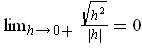    Just as in the proof of Problem 2-4, one can show that, if ch2a82.png were differentiable at 0, then ch2a83.png would be the zero map. On the other hand, by approaching zero along the 45 degree line in the first quadrant, one would then have: ch2a84.png in spite of the fact that the limit is clearly 1.

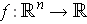  7. Let ch2a85.png be a function such that ch2a86.png . Show that ch2a87.png is differentiable at 0.

    In fact, ch2a88.png by the squeeze principle using ch2a89.png .

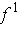  8. Let ch2a90.png . Prove that ch2a91.png is differentiable at ch2a92.png if and only if ch2a93.png and ch2a94.png are, and in this case

    ch2a95.png

    Suppose that ch2a96.png . Then one has the inequality: ch2a97.png . So, by the squeeze principle, ch2a98.png must be differentiable at ch2a99.png with ch2a100.png .

    On the other hand, if the ch2a101.png are differentiable at ch2a102.png , then use the inequality derived from Problem 1-1: ch2a103.png and the squeeze principle to conclude that ch2a104.png is differentiable at ch2a105.png with the desired derivative.

  9. Two functions ch2a106.png are equal up to ch2a107.png order at ch2a108.png if

    ch2a109.png

    1. Show that ch2a110.png is differentiable at ch2a111.png if and only if there is a function ch2a112.png of the form ch2a113.png such that ch2a114.png and ch2a115.png are equal up to first order at ch2a116.png .

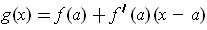      If ch2a117.png is differentiable at ch2a118.png , then the function ch2a119.png works by the definition of derivative.

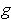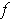      The converse is not true. Indeed, you can change the value of ch2a120.png at ch2a121.png without changing whether or not ch2a122.png and ch2a123.png are equal up to first order. But clearly changing the value of ch2a124.png at ch2a125.png changes whether or not ch2a126.png is differentiable at ch2a127.png .

      To make the converse true, add the assumption that ch2a128.png be continuous at ch2a129.png : If there is a ch2a130.png of the specified form with ch2a131.png and ch2a132.png equal up to first order, then ch2a133.png . Multiplying this by ch2a134.png , we see that ch2a135.png . Since ch2a136.png is continuous, this means that ch2a137.png . But then the condition is equivalent to the assertion that ch2a138.png is differentiable at ch2a139.png with ch2a140.png .

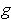    2. If ch2a141.png exist, show that ch2a142.png and the function ch2a143.png defined by

      ch2a144.png

      are equal up to ch2a145.png order at a.

      Apply L'Hôpital's Rule n - 1 times to the limit

      ch2a146.png

      to see that the value of the limit is ch2a147.png . On the other hand, one has:

      ch2a148.png

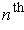      Subtracting these two results gives shows that ch2a149.png and ch2a150.png are equal up to ch2a151.png order at ch2a152.png .