Exercises: Chapter 5, Section 2

  1. Show that ch5b1.png consists of the tangent vectors at ch5b2.png of curves ch5b3.png in ch5b4.png with ch5b5.png .

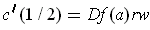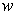    Let ch5b6.png be a coordinate system around ch5b7.png in ch5b8.png ; by replace ch5b9.png with a subset, one can assume that ch5b10.png is a rectangle centered at ch5b11.png . For ch5b12.png and ch5b13.png , let ch5b14.png be the curve ch5b15.png . Then ch5b16.png ranges through out ch5b17.png as ch5b18.png and ch5b19.png vary.

    Conversely, suppose that ch5b20.png is a curve in ch5b21.png with ch5b22.png . Then let ch5b23.png be as in condition ch5b24.png for the point ch5b25.png . We know by the proof of Theorem 5-2, that ch5b26.png is a coordinate system about ch5b27.png where ch5b28.png . Since ch5b29.png , it follows that the tangent vector of ch5b30.png is in ch5b31.png .

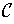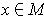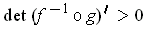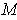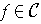  2. Suppose ch5b32.png is a collection of coordinate systems for ch5b33.png such that (1) For each ch5b34.png there is ch5b35.png which is a coordinate system around ch5b36.png ; (2) if ch5b37.png , then ch5b38.png . Show that there is a unique orientation of ch5b39.png such that ch5b40.png is orientation-preserving for all ch5b41.png .

    Define the orientation to be the ch5b42.png for every ch5b43.png , ch5b44.png , and ch5b45.png with ch5b46.png . In order for this to be well defined, we must show that we get the same orientation if we use use ch5b47.png and ch5b48.png . But analogous to the author's observation of p. 119, we know that ch5b49.png implies that

    ch5b50.png

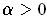    where ch5b51.png . Let ch5b52.png be such that ch5b53.png . Then we have

    ch5b54.png

    i.e. ch5b55.png as desired.

    Clearly, the definition makes ch5b56.png orientation preserving for all ch5b57.png , and this is only orientation which could satisfy this condition.

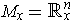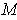  3. If ch5b58.png is an ch5b59.png -dimensional manifold-with-boundary in ch5b60.png , define ch5b61.png as the usual orientation of ch5b62.png (the orientation ch5b63.png so defined is the usual orientation of ch5b64.png . If ch5b65.png , show that the two definitions of ch5b66.png given above agree.

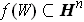    Let ch5b67.png and ch5b68.png be a coordinate system about ch5b69.png with ch5b70.png and ch5b71.png . Let ch5b72.png where ch5b73.png , ch5b74.png and ch5b75.png is perpendicular to ch5b76.png . Note that ch5b77.png is the usual orientation of ch5b78.png , and so, by definition, ch5b79.png is the induced orientation on ch5b80.png . But then ch5b81.png is the unit normal in the second sense.

    1. If ch5b82.png is a differentiable vector field on ch5b83.png , show that there is an open set ch5b84.png and a differentiable vector field ch5b85.png on ch5b86.png with ch5b87.png for ch5b88.png .

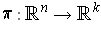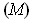      Let ch5b89.png be the projection on the first ch5b90.png coordinates, where ch5b91.png is the dimension of ch5b92.png . For every ch5b93.png , there is a diffeomorphism ch5b94.png satisfying condition ch5b95.png . For ch5b96.png , define ch5b97.png where ch5b98.png . Then ch5b99.png is a differentiable vector field on ch5b100.png which extends the restriction of ch5b101.png to ch5b102.png .

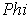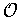      Let ch5b103.png and ch5b104.png be a partition of unity subordinate to ch5b105.png . For ch5b106.png , choose a ch5b107.png with ch5b108.png non-zero only for elements of ch5b109.png . Define

      ch5b110.png

      Finally, let 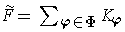ch5b111.png . Then ch5b112.png is a differentiable extension of ch5b113.png to ch5b114.png .

    2. If ch5b115.png is closed, show that we can choose ch5b116.png .

      In the construction of part (a), one can assume that the ch5b117.png are open rectangles with sides at most 1. Let ch5b118.png . Since ch5b119.png is closed, ch5b120.png is compact, and so we can choose a finite subcover of ch5b121.png . We can then replace ch5b122.png with the union of all these finite subcovers for all ch5b123.png . This assures that there are at most finitely many ch5b124.png which intersect any given bounded set. But now we see that the resulting ch5b125.png is a differentiable extension of ch5b126.png to all of ch5b127.png . 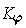In fact, we have now assured that in a neighborhood of any point, ch5b128.png is a sum of finitely many differentiable vector fields ch5b129.png .

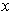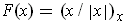      Note that the condition that ch5b130.png was needed as points ch5b131.png on the boundary of the set ch5b132.png of part (a) could have infinitely many ch5b133.png intersecting every open neighborhood of ch5b134.png . For example, one might have a vector field defined on ch5b135.png by ch5b136.png . This is a vector field of outward pointing unit vectors, and clearly it cannot be extended to the point ch5b137.png in a differentiable manner.

  5. Let ch5b138.png be as in Theorem 5-1.
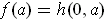    1. If ch5b139.png , let ch5b140.png be the essentially unique diffeomorphism such that ch5b141.png and ch5b142.png . Define ch5b143.png by ch5b144.png . Show that ch5b145.png is 1-1 so that the ch5b146.png vectors ch5b147.png are linearly independent.

      The notation will be changed. Let ch5b148.png , and ch5b149.png be defined, as in the proof of the implicit function theorem, by ch5b150.png ; let ch5b151.png and ch5b152.png . Then ch5b153.png and so ch5b154.png . Also, ch5b155.png . Let ch5b156.png be defined by ch5b157.png . We have changed the order of the arguments to correct an apparent typographical error in the problem statement.

      Now

      ch5b158.png

      which is 1-1 because ch5b159.png is a diffeomorphism. Since it is 1-1, it maps its domain onto a space of dimension ch5b160.png and so the vectors, being a basis, must map to linearly independent vectors.

    2. Show that the orientations ch5b161.png can be defined consistently, so that ch5b162.png is orientable.

      ince ch5b163.png is a coordinate system about every point of ch5b164.png , this follows from Problem 5-10 with ch5b165.png .

    3. If ch5b166.png , show that the components of the outward normal at ch5b167.png are some multiple of ch5b168.png .

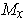      We have ch5b169.png and so by considering the components, we get ch5b170.png This shows that ch5b171.png is perpendicular to ch5b172.png as desired.

  6. If ch5b173.png is an orientable ch5b174.png -dimensional manifold, show that there is an open set ch5b175.png and a differentiable ch5b176.png so that ch5b177.png and ch5b178.png has rank 1 for ch5b179.png .

    Choose an orientation ch5b180.png for ch5b181.png . As the hint says, Problem 5-4 does the problem locally. Further, using Problem 5-13, we can assume locally that the orientation imposed by ch5b182.png is the given orientation ch5b183.png . By replacing ch5b184.png with its square, we can assume that ch5b185.png takes on non-negative values. 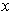So for each ch5b186.png , we have a ch5b187.png defined in an open neighborhood ch5b188.png of ch5b189.png . Let ch5b190.png , ch5b191.png , and ch5b192.png be a partion of unity subordinate to ch5b193.png . 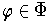Each ch5b194.png is non-zero only inside some ch5b195.png , and we can assume by replacing the ch5b196.png with sums of the ch5b197.png , that the ch5b198.png are distinct for distinct ch5b199.png . Let ch5b200.png be defined by ch5b201.png . Then ch5b202.png satisfies the desired conditions.

  7. Let ch5b203.png be an ch5b204.png -dimensional manifold in ch5b205.png . Let ch5b206.png be the set of end-points of normal vectors (in both directions) of length ch5b207.png and suppose ch5b208.png is small enough so that ch5b209.png is also an ch5b210.png -dimensional manifold. Show that ch5b211.png is orientable (even if ch5b212.png is not). What is ch5b213.png if ch5b214.png is the M"{o}bius strip?

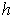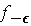    Let ch5b215.png , and ch5b216.png be as in Problem 5-4 in a neighborhood of ch5b217.png . Let ch5b218.png be as in Problem 5-13. Then we have a coordinate systems of the form ch5b219.png and of the form ch5b220.png . Choose an orientation on each piece so that adding ch5b221.png (respectively ch5b222.png ) gives the usual orientation on ch5b223.png . This is an orientation for ch5b224.png .

    In the case of the M"{o}bius strip, the 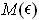ch5b225.png is equivalent to a single ring ch5b226.png .

  8. Let ch5b227.png be as in Theorem 5-1. If ch5b228.png is differentiable and the maximum (or minimum) of ch5b229.png on ch5b230.png occurs at ch5b231.png , show that there are ch5b232.png , such that

    ch5b233.png

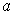    The maximum on ch5b234.png on ch5b235.png is sometimes called the maximum of ch5b236.png subject to the constraints ch5b237.png . One can attempt to find ch5b238.png by solving the system of equations. In particular, if ch5b239.png , we must solve ch5b240.png equations

    ch5b241.png

    in ch5b242.png unknowns ch5b243.png , which is often very simple if we leave the equation ch5b244.png for last. This is Lagrange's method, and the useful but irrelevant ch5b245.png is called a Lagrangian multiplier. The following problem gives a nice theoretical use for Lagrangian multipliers.

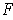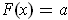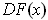    Let ch5b246.png be a coordinate system in a neighborhood of the extremum at ch5b247.png . Then ch5b248.png and so ch5b249.png . Now the image of ch5b250.png is just the tangent space ch5b251.png , and so the row of ch5b252.png is perpendicular to the tangent space ch5b253.png . But we also have ch5b254.png for all ch5b255.png near ch5b256.png , and so ch5b257.png . In particular, this is true at ch5b258.png , and so the rows of ch5b259.png are also perpendicular to ch5b260.png . But, ch5b261.png is of rank ch5b262.png and ch5b263.png is of dimension ch5b264.png , and so the rows of ch5b265.png generate the entire subspace of vectors perpendicular to ch5b266.png . In particular, ch5b267.png is in the subspace generated by the ch5b268.png , which is precisely the condition to be proved.

    1. Let ch5b269.png be self-adjoint with matrix ch5b270.png , so that ch5b271.png . If ch5b272.png , show that ch5b273.png . By considering the maximum of ch5b274.png on ch5b275.png show that there is ch5b276.png and ch5b277.png with ch5b278.png .

      One has

      ch5b279.png

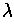      Apply Problem 5-16 with ch5b280.png , so that the manifold is ch5b281.png . In this case, ch5b282.png is a Lagrangian multiplier precisely when ch5b283.png . Since ch5b284.png is compact, ch5b285.png takes on a maximum on ch5b286.png , and so the maximum has a ch5b287.png for which the Lagrangian multiplier equations are true. This shows the result.

    2. If ch5b288.png , show that ch5b289.png and ch5b290.png is self-adjoint.

      Suppose 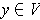ch5b291.png . Then

      ch5b292.png

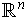      and so ch5b293.png . This shows that ch5b294.png . Since ch5b295.png as a map of ch5b296.png is self-adjoint and ch5b297.png , it is clear that ch5b298.png as a map of ch5b299.png is also self-adjoint (cf p. 89 for the definition).

    3. Show that ch5b300.png has a basis of eigenvectors.

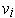      Proceed by induction on ch5b301.png ; the case ch5b302.png has already been shown. Suppose It is true for dimension ch5b303.png . Then apply part (a) to find the eigenvector ch5b304.png with eigenvalue ch5b305.png . Now, ch5b306.png is of dimension ch5b307.png . So, ch5b308.png has a basis of eigenvectors ch5b309.png with eigenvalues ch5b310.png respectively. All the ch5b311.png together is the basis of eigenvectors for ch5b312.png .