be the set of all singular
-cubes, and
the integers. An
-chain is a function
such that
for all but finitely many
.
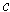Define
and
by
and
.
Show that
and
are
-chains if
and
are. If
, let
also denote the function
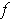such that
and
for
. Show that every
-chain
can be
written
for some integers
and singular
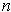-cubes
.
Since
and
, the functions
and
are
-chains if
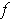and
are.
The second assertion is obvious since
.
and
an integer, define the singular 1-cube
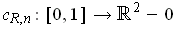by
.
Show that there is a singular 2-cube
such that
.
Define
by
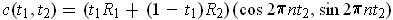where
and
are positive real numbers. The boundary of
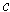is easily seen to
be
.
is a singular 1-cube in
with
,
show that there is an integer
such that
for some
2-chain
.
Given
, let
where
is the function of
Problem 3-41 extended so that it is 0 on the positive
-axis.
Let
so that
is an integer because
.
Define
.
One has
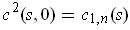and
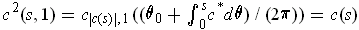. On the other
boundaries,
and
.
So
, as desired.