Exercises: Chapter 3, Section 3

  1. Show that if ch3c1.png are integrable, so is ch3c2.png .

    The set of ch3c3.png where ch3c4.png is not continuous is contained in the union of the sets where ch3c5.png and ch3c6.png are not continuous. These last two sets are of measure 0 by Theorem 3-8; so theee first set is also of measure 0. But then ch3c7.png is integrable by Theorem 3-8.

  2. Show that if ch3c8.png has content 0, then ch3c9.png for some closed rectangle ch3c10.png and ch3c11.png is Jordan-measurable and ch3c12.png .

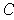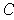    If ch3c13.png has content 0, then it is bounded by Problem 3-9 (a); so it is a subset of an closed rectangle ch3c14.png . Since ch3c15.png has content 0, one has ch3c16.png for some open rectangles ch3c17.png the sum of whose volumes can be made as small as desired. But then the boundary of ch3c18.png is contained in the closure of ch3c19.png , which is contained in the union of the closures of the ch3c20.png (since this union is closed). 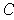But then the boundary of ch3c21.png must be of content 0, and so ch3c22.png is Jordan measurable by Theorem 3-9. Further, by Problem 3-5, one has ch3c23.png which can be made as small as desired; so ch3c24.png .

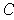  3. Give an example of a bounded set ch3c25.png of measure 0 such that 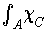ch3c26.png does not exist.

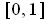    Let ch3c27.png be the set of rational numbers in ch3c28.png . Then the boundary of ch3c29.png is ch3c30.png , which is not of measure 0. So ch3c31.png does not exist by Theorem 3-9.

  4. If ch3c32.png is a bounded set of measure 0 and ch3c33.png exists, show that ch3c34.png .

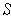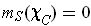    Using the hint, let ch3c35.png be a partition of ch3c36.png where ch3c37.png is a closed rectangle containing ch3c38.png . Then let ch3c39.png be a rectangle of ch3c40.png of positive volume. Then ch3c41.png is not of measure 0 by Problem 3-8, and so ch3c42.png . But then there is a point of ch3c43.png outside of ch3c44.png ; so ch3c45.png . Since this is true of all ch3c46.png , one has ch3c47.png . Since this holds for all partitions ch3c48.png of ch3c49.png , it follows that ch3c50.png if the integral exists.

  5. If ch3c51.png is non-negative and ch3c52.png , show that ch3c53.png has measure 0.

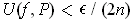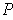    Following the hint, let ch3c54.png be a positive integer and ch3c55.png . Let ch3c56.png . Let ch3c57.png be a partition of ch3c58.png such that ch3c59.png . Then if ch3c60.png is a rectangle of ch3c61.png which intersects ch3c62.png , we have ch3c63.png . So ch3c64.png . By replacing the closed rectangles ch3c65.png with slightly larger open rectangles, one gets an open rectangular cover of ch3c66.png with sets, the sum of whose volumes is at most ch3c67.png . So ch3c68.png has content 0. Now apply Theorem 3-4 to conclude that ch3c69.png has measure 0.

  6. Let ch3c70.png be the open set of Problem 3-11. Show that if ch3c71.png except on a set of measure 0, then f is not integrable on ch3c72.png .

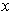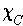    The set of ch3c73.png where ch3c74.png is not continuous is ch3c75.png which is not of measure 0. If the set where ch3c76.png is not continuous is not of measure 0, then ch3c77.png is not integrable by Theorem 3-8. On the other hand, if it is of measure 0, then taking the union of this set with the set of measure 0 consisting of the points where ch3c78.png and ch3c79.png differ gives a set of measure 0 which contains the set of points where ch3c80.png is not continuous. So this set is also of measure 0, which is a contradiction.

  7. Show that an increeasing function ch3c81.png is integrable on ch3c82.png .

    This is an immediate consequence of Problem 3-12 and Theorem 3-8.

  8. If ch3c83.png is a closed rectangle, show that ch3c84.png is Jordan measurable if and only if for every ch3c85.png there is a partition ch3c86.png of ch3c87.png such that ch3c88.png , where ch3c89.png consists of all subrectangles intersecting ch3c90.png and ch3c91.png consists of allsubrectangles contained in ch3c92.png .

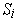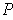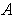    Suppose ch3c93.png is Jordan measurable. Then its boundary is of content 0 by Theorem 3-9. Let ch3c94.png and choose a finite set ch3c95.png for ch3c96.png of open rectangles the sum of whose volumes is less than ch3c97.png and such that the ch3c98.png form a cover of the boundary of ch3c99.png . Let ch3c100.png be a partition of ch3c101.png such that every subrectangle of ch3c102.png is either contained within each ch3c103.png or does not intersect it. This ch3c104.png satisfies the condition in the statement of the problem.

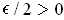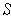    Suppose for every ch3c105.png , there is a partition ch3c106.png as in the statement. Then by replacing the rectangles with slightly larger ones, one can obtain the same result except now one will have ch3c107.png in place of ch3c108.png and the ch3c109.png will be open rectangles. This shows that the boundary of ch3c110.png is of content 0; hence ch3c111.png is Jordan measurable by Theorem 3-9.

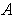  9. If ch3c112.png is a Jordan measurable set and ch3c113.png , show that there is a compact Jordan measurable set ch3c114.png such that ch3c115.png .

    Let ch3c116.png be a closed rectangle containing ch3c117.png . Apply Problem 3-21 with ch3c118.png as the Jordan measurable set. Let ch3c119.png be the partition as in Problem 3-21. Define ch3c120.png . Then ch3c121.png and clearly ch3c122.png is Jordan measurable by Theorem 3-9. Further ch3c123.png .